Algebraic Geometry Conference

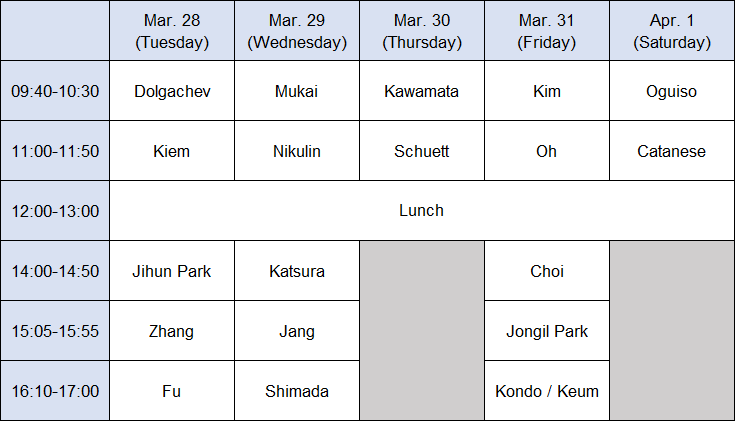
Tuesday (March 28)

09:40-10:30 Igor Dolgachev
"The decomposition and the inertia subgroups of a rational curve in a group of birational automorphisms of an algebraic surface"

Given algebraic surface S and an irreducible curve C on it, one asks about the group of birational automorphisms of the pair (S,C) and its subgroup that fixes C pointwise. I will discuss a few known examples when C is a smooth rational curve and the latter group contains an element of positive entropy. Apparently, the first example was known to A. Coble, although he did not state it explicitly. A new example was found recently by John Lesieutre. I will discuss an example of a group of automorphisms of a rational or a K3 surface that is isomorphic to a non-elementary discrete group of isometries of a hyperbolic space that leaves a smooth rational curve invariant and hence admits a non-trivial homomorphism to the group of Moebius transformations. The problem of injectivity of this map is related to the problem of freeness of some rotation group associated with a regular tetrahedron.

11:00-11:50 Young-Hoon Kiem
“Intersection theory - old and new”

I will speak about a history of enumerative geometry and intersection theory as well as recent advances in virtual intersection theory and effective techniques to handle virtual fundamental classes with applications.

14:00-14:50 Jihun Park
"Asymptotic invariants and K-stability of Fano varieties"

Since entering the 21st century we have witnessed dramatic developments in the study of the Yau-Tian-Donaldson conjecture concerning the existence of Kaehler-Einstein metrics on Fano manifolds and stability. The challenge to the conjecture has been highlighted by Chen, Donaldson, Sun and Tian who have completed the proof of the following celebrated statement:

Theorem. A smooth Fano manifold admits a Kaehler-Einstein metric if and only if it is K-polystable with respect to the anticanonical polarization.

There are not so many results concerning K-stability of specified smooth Fano varieties, not deduced by Kaehler-Einstein metrics. It seems almost infeasible to consider all possible degenerations of a given Fano manifold. Even for del Pezzo surfaces, we do not have complete description of their degenerations. In this talk, I review several invariants that enable us to have a detour studying K-stability of Fano manifolds.

15:05-15:55 De-Qi Zhang
"Rationality of homogeneous varieties"

When G is a linear algebraic group and H a closed connected subgroup, we show that X = G/H is a rational variety provided that either dim X is at most 10 and the ground field k = C, or rank H = rank G and the semi simple quotient of G is isogenous to a product of those of Dynkin type A, or type C (when char k is not 2). This is a joint work with C. Chin.

16:10-17:00 Baohua Fu
"In Search of Isolated Symplectic Singularities"

An isolated symplectic singularity is either an ADE surface singularity or a normal isolated singularity of dimension at least four whose smooth part carries a symplectic form. Previous known examples are nite quotient of C2n or minimal nilpotent orbit closures in simple Lie algebras. These singularities have strong relationship with for example the LeBrun-Salomon conjecture on Fano contact manifolds. I'll report on our attempts (joint with D. Juteau, P. Levy and E. Sommers) in search of new isolated symplectic singularities.


Wednesday (March 29)

09:40-10:30 Shigeru Mukai
"Enriques surfaces with rational curves of Petersen configuration"

The famous Petersen graph P10 appears most easily as the dual graph of 10 lines on a quintic del Pezzo surface in algebraic geometry. There are two types of Enriques surfaces similar to this. One is covered by a Hessian quartic surface and was studied in detail by Dolgachev-Keum(2002). In this talk I discuss about another type of Enriques surfaces with 15 rational curves whose dual graph is L(P10), the line graph of P10.

11:00-11:50 Viacheslav Nikulin
"Examples of lattice-polarized K3 surfaces with automorphic discriminant, and Lorentzian Kac--Moody algebras"

Using our results about Lorentzian Kac--Moody algebras and arithmetic mirror symmetry, we give examples of lattice-polarized K3 surfaces with automorphic discriminant. See arXiv:1702.07551 for some details. These are joint results with Valery Gritsenko.

14:00-14:50 Toshiyuki Katsura
“Classification of Enriques surfaces with finite automorphism groups in characteristic 2”

We consider Enriques surfaces with finite automorphism groups in characteristic 2, and by using the configurations of nodal curves we give the complete classification of them. We have 3 types of singular Enriques surfaces, 5 types of supersingular Enriques surfaces and 8 types of classical Enriques surfaces. Note that in characteristic 0 we have 7 types of Enriques surfaces with finite automorphism groups. We will also examine the structure of automorphism groups. This is a joint work with S. Kondo and G. Martin.

15:05-15:55 Junmyeong Jang
"Non-symplectic index of supersingular K3 surfaces"

The non-symplectic index of an algebraic K3 surface is the order of the image of the representation of the automorphism group of the K3 surface on the global two forms. If the base field is the complex field, the non-symplectic index is finite and its Euler phi value is at most 20. If the base field is of positive characteristic and the K3 surface is of finite height, we have a similar result through a lifting argument. In this talk, we calculate the non-symplectic index of all supersingular K3 surfaces over a field of characteristic at least 5 using the crystalline Torelli theorem.

16:10-17:00 Ichiro Shimada
"On an Enriques surface associated with a quartic Hessian surface"

We present several algorithmic methods to investigate the geometry of a complex Enriques surface by computation on the Néron-Severi lattices of the Enriques surface and of its covering K3 surface. We apply them to an Enriques surface Y whose étale double cover X is birational to a general quartic Hessian surface. Using the result on the automorphism group of X due to Dolgachev and Keum, we obtain a finite presentation of the automorphism group of Y. This automorphism group is compared with the automorphism group of the generic Enriques surface. A fundamental domain of the action of the automorphism group on the nef cone of Y is described explicitly. The list of elliptic fibrations on Y and the list of combinations of rational double points that can appear on a surface birational to Y are presented.

 
Thursday (March 30)

09:40-10:30 Yujiro Kawamata
"Derived McKay correspondence for GL(3,C)"

I will consider the consequence of the change of birational models to the change of derived categories by looking at the change of canonical divisors. I will consider the case of 3-dimensional quotient singularities and their maximal resolutions.

11:00-11:50 Matthias Schuett
"Configurations of nodal curves on Enriques surfaces"

Following work of Keum and collaborators, we will study Enriques surfaces admitting interesting configurations of nodal curves. Throughout we will try to cover all characteristics, including characteristic two. Time permitting, we will sketch an application to Enriques surfaces with everywhere good reduction.


Friday (March 31)

09:40-10:30 Bumsig Kim
"Quasimap Wall-crossings and Mirror Symmetry"

We present a wall-crossing formula for the virtual classes of ε-stable quasimaps to GIT quotients and sketch its proof for complete intersections in projective space, with no positivity restrictions on their first Chern class.

As a consequence, the wall-crossing formula relating the genus g descendant Gromov-Witten potential and the genus g ε-quasimap descendant potential is established.

For the quintic threefold, our results may be interpreted as giving a rigorous and geometric interpretation of the holomorphic limit of the BCOV B-model partition function of the mirror family.

This is a joint work with I. Ciocan-Fontanine.

11:00-11:50 Yong-Geun Oh
"Lagrangian Floer theory of Gelfand-Cetlin systems"

In this lecture, I will explain the study of nondisplaceability of Lagrangian fibers of the Gelfand-Cetlin systems by extending Fukaya-Oh-Ohta-Ono’s study of toric cases to the complex partial flag manifolds. This also generalizes the previous study of Nishino-Nohara-Ueda and Nohara-Ueda. I will particularly explain construction of a continuum family of nondisplaceable Lagrangian tori degenerating to the three-sphere in the full flag manifold F(1,2,3). This also proves the limiting Lagrangian sphere is nondisplaceable, the question of which was left open in Nohara-Ueda’s earlier study. This talk is based on the joint work with Yunhyung Cho and Yoosik Kim.

14:00-14:50 Sung Rak Choi
“Chamber decomposition of psedueoffective cones”

We introduce two decompositions of the pseudoeffective cone into the chambers defined by the asymptotic base loci and Zariski decompositions, respectively. We show that on a Mori dream space, the two decompositions precisely coincide and the decomposition is locally finite.

15:05-15:55 Jongil Park
"A note on rational blowdown surgery"

Since gauge theory was introduced in 1982, people working on 4-manifolds have developed various techniques and surgeries and they have obtained many fruitful and remarkable results on 4-manifolds in last 35 years. Among them, a rational blowdown surgery technique initially introduced by R. Fintushel and R. Stern and later generalized by J. Park turned out to be one of the simple but powerful techniques to construct a new family of 4-manifolds.

In this talk, first I'd like to briefly review what we have obtained by using a rational blowdown surgery. And then I'll explain in some details that any minimal symplectic filling of the link of a quotient surface singularity equipped with the canonical contact structure can be obtained by a sequence of rational blowdowns and blowing-ups from the minimal resolution the corresponding quotient surface singularity.

16:10-17:00 Shigeyuki Kondo / JongHae Keum

Saturday (April 1)

09:40-10:30 Keiji Oguiso
"A criterion for the primitivity of a birational automorphism of a Calabi-Yau manifold and an application"

A biraional automorphism of a projective manifold is said to be primitive if it admits no non-trivial equivariant rational dominant map to a lower dimensional projective manifold. We shall give a sufficient condition on the primitivity of a birational automorphism of a Calabi-Yau manifold in purely algebro-geometric terms. As an application, we shall give an explicit construction of Calabi-Yau manifolds of Picard number 2 of any dimension greater than 2, with primitive birational automorphisms of first dynamical degree greater than one.

11:00-11:50 Fabrizio Catanese
"Group representations and canonical surfaces of very high degree"

Given a surface S of general type, we denote by d the degree of the canonical image. d is bounded by the canonical volume K^2, and by the BMY inequality we have d ≤ 9χ.

In practice, what is the maximum d for p_g=4,5,6? Up to now, the best lower bound is quite low (in joint work with Ingrid Bauer we achieved ball quotients with p_g=4, K^2 = 45, but the record d for p_g=4 is still 28).

For p_g=6, where one expects to have surfaces whose canonical map is an embedding, there are interesting ties with methods and questions of homological algebra (Walter's bundle Pfaffians), which led to the question whether 18 would be the upper bound (I answered by getting degree 24). I recently constructed several connected components of the moduli space, of surfaces S of general type with p_g = 5,6 whose canonical map has image Σ of very high degree, d=48 for p_g =5, d=56 for p_g =6. I shall discuss these and other results, based on the theory of surfaces isogenous to a product of curves, S = (C1 × C2)/G. Concerning the canonical map of these surfaces, and of Galois covers, representation theory then enters the picture through several questions, the easiest one being: when does a submodule V of the group algebra ℂ[G] yield a projective embedding of G?