First Encounter to Quantum Topology: School and Workshop
Date: Jul. 15 ~ Jul. 18 (School), Jul. 20 ~ Jul. 22 (Workshop), 2015
Place: Rm. 1503, KIAS, Seoul, Korea
|

| Title & Abstract | Home > Title & Abstract |
File : Presentation files.zip
Speaker: Jinseok Cho (PMI, POSTECH) Title: Reidemeister transformations of the potential function and the solution
Abstract: To get the hyperbolic structure of a knot complement, we need to find a good triangulation of the knot complement and to solve the hyperbolicity equations. Usually this triangulation is determined by the knot diagram. Therefore, if we change the diagram by Reidemeister moves, then the triangulation changes and we have to solve the equations again. In this talk, we introduce a new method to find a solution combinatorially from the knot diagram using special types of quandle proposed by Inoue and Kabaya. This solution changes naturally under the Reidemeister moves so that we can keep track of the solution under the change of diagram. This method is motived by the optimistic limit of the colored Jones polynomial, which is a physical interpretation of the volume conjecture. This is joint work with Jun Murakami.
Speaker: Oliver Dasbach (Louisiana State University)
Title 1: On the Turaev surface
Abstract 1: Turaev constructed from a knot diagram an orientable surface on which the knot projects. The diagram of the knot on the Turaev surface can be encoded by a ribbon graph, and the Jones polynomial and Khovanov homology can be computed from it. Moreover, the genus of the Turaev surface gives bounds on the support of knot homologies, and on the signature of a knot. We will give an introduction to some properties of the Turaev surface, and state recent results.
Title 2: Coefficients of the colored Jones polynomial, and augmented links
Abstract 2: We compare properties of the hyperbolic volume of augmented links with properties of the colored Jones polynomial. In particular we study the behavior under certain generalizations of the connected sum. (This is joint work with Mustafa Hajij.)
Title 1: On the Turaev surface
Abstract 1: Turaev constructed from a knot diagram an orientable surface on which the knot projects. The diagram of the knot on the Turaev surface can be encoded by a ribbon graph, and the Jones polynomial and Khovanov homology can be computed from it. Moreover, the genus of the Turaev surface gives bounds on the support of knot homologies, and on the signature of a knot. We will give an introduction to some properties of the Turaev surface, and state recent results.
Title 2: Coefficients of the colored Jones polynomial, and augmented links
Abstract 2: We compare properties of the hyperbolic volume of augmented links with properties of the colored Jones polynomial. In particular we study the behavior under certain generalizations of the connected sum. (This is joint work with Mustafa Hajij.)
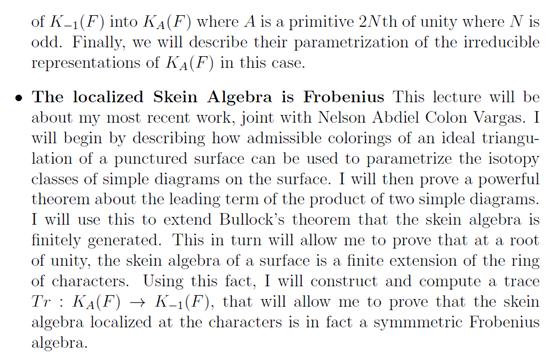
Speaker: Charles Frohman (University of Iowa)
Title & Abstract:


Title & Abstract:



Speaker: Tetsuya Ito (Kyoto University)
Title 1: Braid groups representation in quantum topology, topological overview
Abstract 1: In this talk we give an overview of the role of representations of the braid groups in quantum topology, emphasizing topological aspect. We explain how finite type invariants and Kontsevich invariant of braids appear in the context of (classical) topology. If time permits, we will also discuss how the Burau representation, the most fundamental braid group representation, appears in the context of quantum representation.
Title 2: Homological representation formula of some quantum sl_2 invariants
Abstract 2: Roughly speaking, there are two ways to construct linear representations of the braid groups.The first one is homological, based on the identification of the braid group as the mapping class group of punctured disc, and the second one is based on quantum groups and has more algebraic flavor.
In this talk after reviewing a connection between homological and quantum representations, we give a formula of some quantum sl_2 invariants in terms of homological representation. We hope that this helps us to understand the topological content of quantum invariants.
Speaker: Christine Lee (Michigan State University)
Title: The colored Jones polynomial of non semi-adequate links
Abstract: A semi-adequate link admits a diagram which is either A- or B- adequate. The colored Jones polynomial of semi-adequate links has been shown to have properties which directly relate to the topology and the geometry of the link complement. For example, the polynomial exhibits a stability behavior and has a "tail" for A-adequate links. The Slope Conjecture is true for A-adequate links, and certain stable coefficients forming the tail give volume bounds for the hyperbolic knot complement. In this talk I will discuss some results and potential problems in this direction for links which are not semi-adequate. The first is a stability result and the definition of a "tail" for all links, and the second result, joint with R. van der Veen, is that the Slope Conjecture is true for a new family of 3-string pretzel knots.
Speaker: Sangmin Lee (Seoul National University)
Title: Holography of 3d-3d correspondence at Large N
Abstract: We study the physics of multiple M5-branes compactified on a hyperbolic 3-manifold. On the one hand, it leads to the 3d-3d correspondence which maps a 3d, N=2 superconformal field theory to a pure Chern-Simons theory on the 3-manifold. On the other hand, it leads to a warped AdS4 geometry in M-theory holographically dual to the superconformal field theory. Combining the holographic duality and the 3d-3d correspondence, we propose a conjecture for the large N limit of the perturbative free energy of a Chern-Simons theory on hyperbolic 3-manifold. The conjecture claims that the tree, one-loop and two-loop terms all share the same N^3 scaling behavior and are proportional to the volume of the 3-manifold, while the three-loop and higher terms are suppressed at large N. Under mild assumptions, we prove the tree and one-loop parts of the conjecture. For the two-loop part, we test the conjecture numerically in a number of examples and find precise agreement. We also confirm the suppression of higher loop terms in a few examples.
[Remarks]
(1) This talk will be based on a recent paper with the same title: D. Gang, N. Kim, S. Lee, JHEP 1504 (2015) 091.
(2) The paper was written from physicists' point of view, but the talk will be prepared such that mathematicians in related fields can understand the main results.
Speaker: Hitoshi Murakami (Tohoku University)
Title 1: An introduction to the volume conjecture and its generalizations, I, II, III, IV
Title 2: The volume conjecture for non-simple knots
Abstract 2: We will study a relation of the colored Jones polynomial of a non-simple knot to the Chern-Simons invariant and the Reidemeister torsion.
Speaker: Peter Samuelson (University of Toronto)
Title: Skein theory, the torus, and Hecke algebras
Abstract: Many quantum knot invariants can be computed using "skein relations," which allow one to switch a crossing in a knot diagram at the cost of "lower order terms." The space of links in a thickened surface modulo the skein relations is an algebra, where the multiplication is given by stacking. If the surface is a torus, then it turns out that this algebra is a specialization of the double affine Hecke algebra, which is a quantum algebra related to Macdonald polynomials. We will discuss both of these algebras and their relationship.
Speaker: Ralf Schiffler (University of Connecticut)
Title: Cluster algebras and Chern-Simons invariants
Abstract: Hikami and Inoue have established a relation between the complex volume of knots and a particular kind of cluster algebras. The computation of Chern-Simons invariants can be reduced to solving a system of equations in the cluster algebra. The cluster algebras that appear in this context are those associated to a disk with m punctures and 4 marked points on the boundary.
In the first talk we shall give a general definition of cluster algebras using this particular type as a running example. In the second talk, we shall discuss how to make actual computations in the cluster algebra using combinatorial formulas in terms of perfect matchings of so-called snake graphs.
Speaker: Roland van der Veen (University of Amsterdam)Title: Invitation to the quantum plane
Abstract: Coordinates on the plane are two functions x,y that commute: xy=yx and form an algebra, say polynomials in two variables, C[x,y].
It has many symmetries including the linear ones given by the 2x2 matrices GL(2) and perhaps SL(2) and SU(2). For example all irreducible representations of SU(2) can be realized as homogeneous polynomials in x and y.
What if we had coordinates that q-commute instead? So q xy = xy. Our functions x,y now can be viewed as coordinates on the quantum
plane and its symmetries form the (matrix) quantum group GL_q(2), SL_q(2) and SU_q(2). They are dual to the more usual quantum groups. I will argue how this leads to a different perspective on many objects in quantum topology, including spin networks, representation varieties and the colored Jones polynomial.
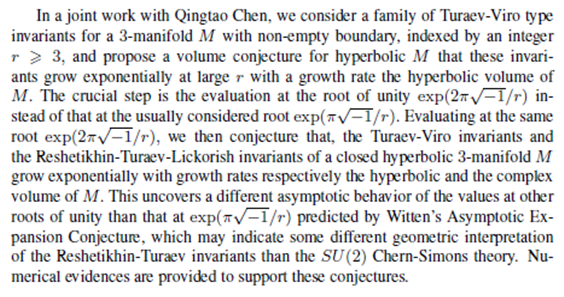
Speaker: Tian Yang (Stanford University)
Title: Turaev-Viro invariants and a volume conjecture
Abstract: