▶ Title: Families of Segre fourfolds with a view to del Pezzo fibrations

Jinwon Choi

▶ Title: Log BPS numbers: motivation, definition and conjectures

▶ Abstract: We propose a new curve counting invariant from log geometry of del Pezzo surfaces. The log BPS numbers is motivated by a numerical property of the genus zero local BPS invariants. We present a rigorous definition of the log BPS numbers via Gromov-Witten theory and formulate a conjecture which relates the local and log BPS numbers. This is joint work with Michel van Garrel, Sheldon Katz and Nobuyoshi Takahashi.

Sung Rak Choi

▶ Title: On a generalization of the Cone Theorem

▶ Abstract: The Cone Theorem describes the Mori cone in the $K_X$-negative part and it is well known that the theorem played a crucial role in the development of the minimal model program. Batyrev conjectured that the cone of movable curves also has similar structure and the recent breakthrough of the bounded of Fano varieties is expected to confirm the conjecture. In this talk, we discuss a possible alternative approach to the conjecture and cosider a generalization of the conjecture. This is an on-going joint work with Yoshinori Gongyo


Kiryong Chung

▶ Title: Geometry of moduli spaces of rational curves in linear sections of Grassmannian

▶ Abstract: In this talk, I will prove that the spaces of rational curves of degree at most 3 in linear sections of the Grassmannian Gr(2, 5) are all rational varieties. 
     I also study their compactifications and birational geometry. This is joint work with J. Hong and S. Lee.

Ciro Ciliberto

▶ Title: Curves on general hypersurfaces in projective space
▶ Abstract: This talk concerns the existence of curves with low gonality on smooth hypersurfaces $Xsubset mathbb{P}^{n+1}$. 
     After reviewing a series of results on this topic, I will report on a recent progress on the subject in collaboration with F. Bastianelli, F. Flamini and P. Supino. In particular, we obtained that if $Xsubset mathbb{P}^{n+1}$ is a very general hypersurface of degree $dgeqslant 2n+2$, the least gonality of a curve $Csubset X$ passing through a general point of $X$ is $gon(C)=d-leftlfloorfrac{sqrt{16n+1}-1}{2}rightrfloor$, apart from some exceptions we list.

Luis E. Solá Conde

▶ Title: Uniform flag bundles

▶ Abstract: It is known since the 1980s that low rank uniform vector bundles on projective spaces are homogeneous. Whilst this fact can be naturally extended to the case of uniform vector bundles on (some) rational homogenous spaces, the results obtained up to date seem to indicate that the problem should be considered in the setting of principal G-bundles (G semisimple) on Fano varieties. In this talk we will present some basic facts on the topic, present some conjectures, and establish certain splitting conditions.

Kangjin Han

▶ Title: Syzygetic characterization of ACM varieties with d-linear resolution

▶ Abstract: Given a closed variety X in a projective space, the Betti table of X, B(X), which comes from the minimal free resolution of the homogeneous coordinate ring, tells us many interesting algebraic and geometric properties of X in its embedding. Since the foundational paper by Green had appeared, the first non-trivial linear strand part in the Betti table, arising from defining equations of degree 2, has been intensively studied and focused by many people during the past decades. 
     In this talk, we consider the $d$-th linear strand of B(X) for $dgeq 3$. We first describe some geometric meanings of a syzygy condition N_{d,p}, and obtain degree upper bounds as a consequence. In particular, we prove that the equality holds if and only if X is arithmetically Cohen-Macaulay with d-linear resolution. Next, we introduce a new notion ND(k) to understand the (k+1)-th strand of the Betti table and raise many interesting questions similar to the previous quadratic case (k=1). We report some progress on these questions. This is a joint work with J. Ahn and S. Kwak.

Sukmoon Huh

▶ Title: Representation type of surfaces in three-dimensional projective space

▶ Abstract:: The category of indecomposable arithmetic Cohen-Macaulay(aCM) sheaves supported on a given projective variety is believed to measure the complexity of the variety. Following the trichotomy in representaiton theory, a classification of aCM variety was proposed as finite, tame or wild type. Recently the trichotomy was proven to be exhaustive for the aCM varieties that are not a cone. In this talk we report our recent result proving that every surface with a regular point in the three-dimensional projective space of degree at least four, is of wild representation type under some condition. Indeed, we construct arbitrarily large dimensional families of indecomposable pairwise non-isomorphic aCM vector bundles. 
     This is a joint work with Edoardo Ballico.

DongSeon Hwang

▶ Title: SSDB: $alpha$-version

▶ Abstract: The Graded Ring Database(GRDB) plays an important role in the experimental investigation on Fano or Calabi-Yau varieties. We would like to launch a website aiming at providing experimental computations on singular surfaces by the end of 2018. Currently, we are launching only alpha test version which includes some new information on log del Pezzo surfaces of Picard number one.  
     In this talk, basic features of the website will be demonstrated after presenting an introduction to the concept of cascades of log del Pezzo surfaces of Picard number one.

Junmyeong Jang

▶ Title: Non-symplectic index of K3 surfaces over odd characteristic

▶ Abstract:  The non-symplectic index of an algebraic K3 surface is the order of the image of the representation of the automorphism group of the K3 surface on the global two forms. If the base field is the complex field, the non-symplectic index is finite and its Euler phi value is at most 20. In this talk, we will see if the base field is of odd characteristic and the K3 surface is of finite height, we have a similar result through a lifting argument. Also we calculate the non-symplectic index of all supersingular K3 surfaces over a field of characteristic at least 5 using the crystalline Torelli theorem. 

Emanuele Macrì

▶ Title: Bridgeland stability and the genus of space curves

▶ Abstract: I will give an introduction to various notions of stability in the bounded derived category of coherent sheaves on the three-dimensional projective space. 
     As application I will show how to use these techniques towards the study of space curves.
     This is joint work in progress with Benjamin Schmidt.

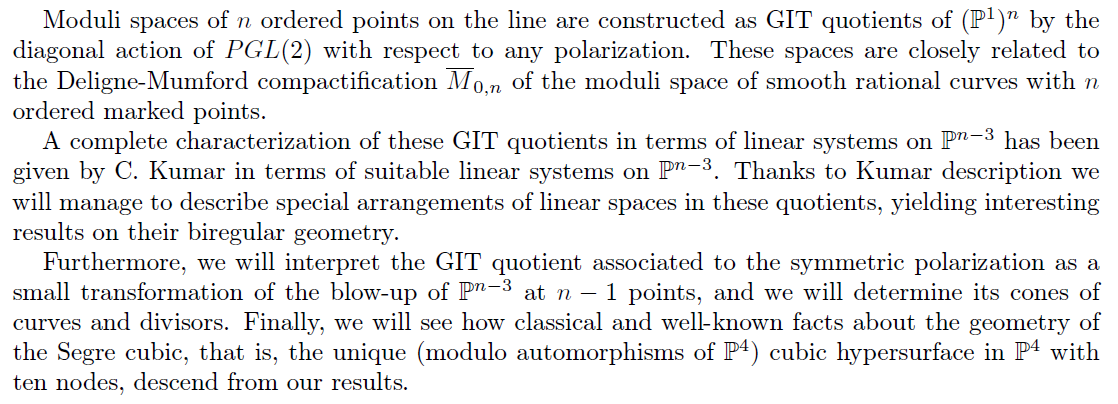
▶ Title: On the birational geometry of moduli spaces of points on the line

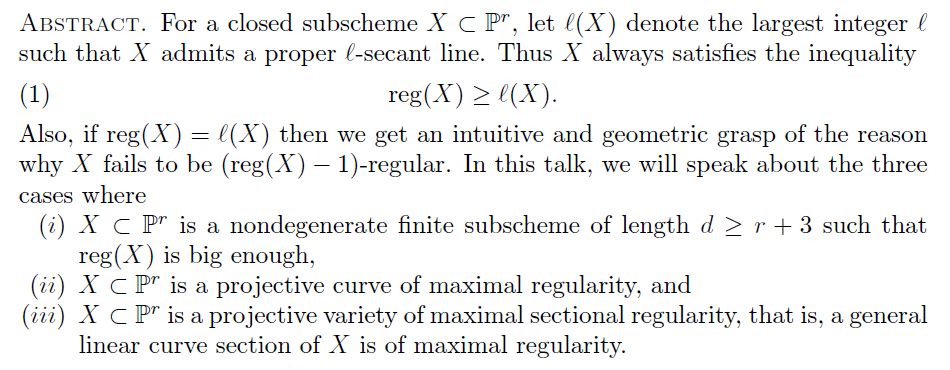
▶ Title: Regularity and multisecant lines

▶ Title: A Castelnuovo-Mumford regularity bound for threefolds with rational singularities

▶ Abstract: The Castelnuovo-Mumford regularity of an embedded projective variety is the maximal degree of generators of all syzygies of the defining ideal, so in particular it gives an upper bound for degree of defining equations. Eisenbud-Goto's regularity conjecture predicts a sharp bound for Castelnuovo-Mumford regularity of a projective variety, and it has been verified for lower dimensional cases. However, McCullough-Peeva recently constructed counterexamples to this conjecture. One of their counterexamples is a threefold in P5 with one dimensional singular locus. The major obstruction to bounding regularity may be the bad singularities on a variety. Thus it is natural to expect that if a variety had mild singularities then some good bound for regularity would exist. In this talk, we prove a Castelnuovo-Mumford regularity bound for threefolds with rational singularities. Note that a threefold with rational singularities could have one dimensional singular locus. To achieve the main result, we analyze the Loewy length of fibers of a general projection and the dimension of the variety swept out by secant lines. This is joint work with Wenbo Niu.

▶ Title: Congruences of 5--secant conics and the rationality of some admissible cubic fourfolds

▶ Abstract: Kuznetsov Conjecture and the work of Hassett predict that a general cubic fourfold belonging to an irreducible divisor $mathcal C_d$ parametrizing smooth cubic hypersurfaces in $P^5$ of discriminant $d$ is rational if and only if $d$ is an admissible value in the sense of Hassett, that is, if and only if $d>6$ is an even integer not divisible by 4, by 9 nor by any odd prime of the form $2+3m$. 
     Our main result is the proof of this conjecture for the smallest admissible values $d=26$ and $d=38$ (the case $d=14$ being classical), via the construction of a {it congruence of 5-secant conics} to a surface $S_d$ contained in the general element of $mathcal C_d$ for $d=14,26,38$. 
     This is joint work with Giovanni Staglian’ o. 

▶ Title: Complex Ball quotients and hyperkähler fourfold

▶ Abstract: In a famous paper of 2011 Allcock, Carlson and Toledo describe the moduli space of smooth cubic threefolds as a 10-dimensional ball quotient. We show how the 10-dimensional ball quotient can also be described as the moduli space of certain hyperkähler fourfolds with a non-symplectic automorphism of order three. 
     We then completely describe the hyperkähler fourfolds and we identify them with the Fano variety of lines of cubic fourfolds that are triple covers of the 4 dimensional complex projective space ramified on a smooth cubic threefold. 
     We finally describe degenerations of the hyperkähler fourfolds which are related to degenerations of the smooth cubic threefolds to the nodal and the chordal locus in the complex ball quotient. 
     This is a joint work with S. Boissière and C. Camere

▶ Title: Deformations of normal surface singularities with C^*-actions

▶ Abstract: We classify all deformations of normal surface singularities admitting C^*-actions by applying the so-called controlled minimal model program for 3-folds. This is a joint work in progress with Heesang Park.

▶ Title: Bridgeland stability for semiorthogonal decompositions and cubic fourfolds

▶ Abstract: We illustrate a new method to induce stability conditions on semiorthogonal decompositions and apply it to the Kuznetsov component of the derived category of cubic fourfolds. We use this to reprove the Torelli theorem for cubic fourfolds, to generalize the results of Addington-Thomas and to study the reach hyperkaehler geometry associated to these hypersurfaces. This is the content of joint works with Arend Bayer, Howard Nuer, Marti' Lahoz, Emanuele Macri' and Alex Perry.

▶ Title: On the boundedness of Calabi-Yau varieties in low dimension

▶ Abstract: I will discuss new results towards the birational boundedness of low-dimensional elliptic Calabi-Yau varieties, joint work with Gabriele Di Certo. Recent work in the minimal model program suggests that pairs with trivial log canonical class should satisfy some boundedness properties. I will show that 4-dimensional Calabi-Yau pairs which are not birational to a product are indeed log birationally bounded. This implies birational boundedness of elliptically fibered Calabi-Yau manifolds with a section, in dimension up to 5. If time allows, I will also try to discuss a first approach towards boundedness of rationally connected CY varieties in low dimension (joint with G. Di Cerbo, W. Chen, J. Han and, C. Jiang).

▶ Title: On the cone of effective cycles on the symmetric products of curves

▶ Abstract: I will report on a joint work with F. Bastianelli, A. Kouvidakis and A. F. Lopez in which we study the cone of (pseudo-)effective cycles on symmetric products of a curve. 
     We first prove that the diagonal cycles span a face of the pseudo-effective cone of cycles in any given dimension. Secondly, we look at the contractibility faces associated to the Abel-Jacobi morphism towards the Jacobian and in many cases we are able to compute their dimension. The classicial Brill-Nother theory will of course play a special role in our analysis.