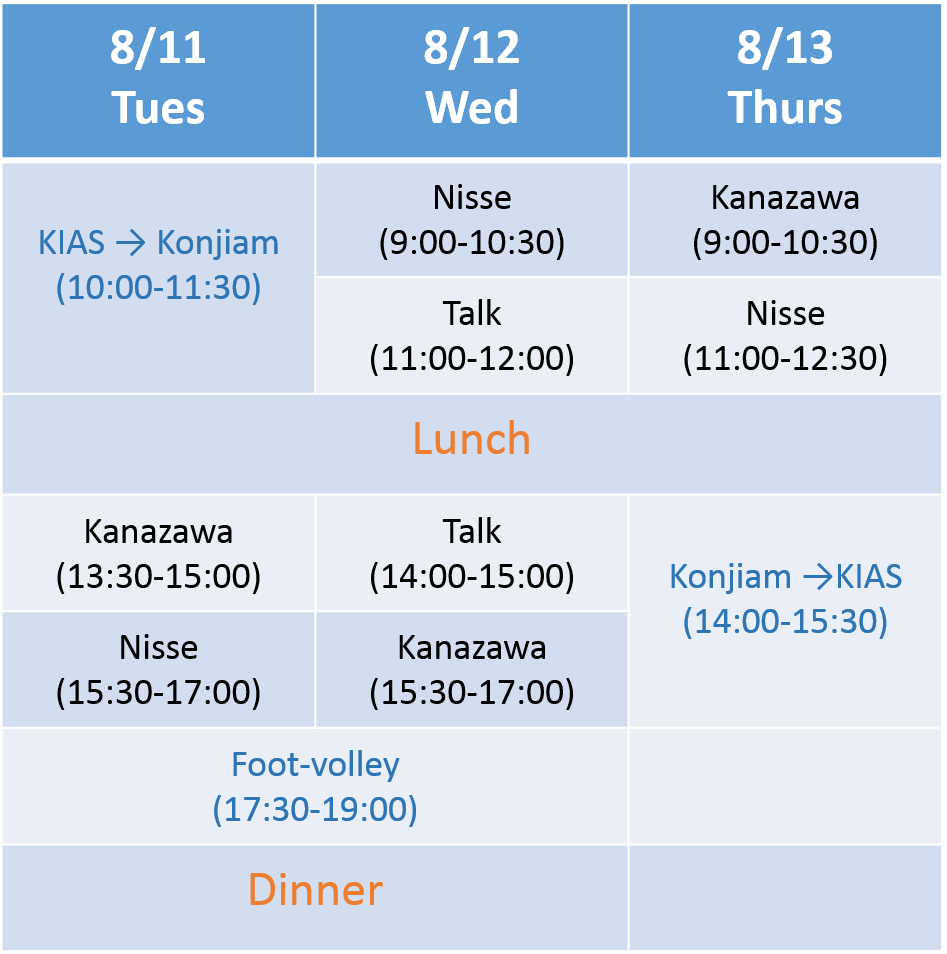
1. Kanazawa

- Title: Lectures on BCOV holomorphic anomaly equations
- Abstract: This lecture is a gentle introduction to the higher genus mirror symmetry, with emphasis on the BCOV holomorphic anomaly equations. Mirror symmetry is, roughly put, a certain duality between symplectic geometry (A-model) and complex geometry (B-model) among Calabi--Yau manifolds. For example, the classical genus 0 mirror symmetry is about a duality between the genus 0 Gromov--Witten theory and the Hodge theory. I will explain how this generalizes to the higher genus cases, introducing a profound recursive structure. I will also compare the theory with the Kaneko-Zagier theory of quasi-modular forms.

- References:
(1) Lectures on BCOV Holomorphic AnomalyEquations, http://arxiv.org/abs/1409.4105
(2) BCOV ring and holomorphic anomaly equation, http://arxiv.org/abs/0810.4795
(3) Higher genus Gromov-Witten invariants of the Grassmannian and the Pfaffian Calabi-Yau threefolds http://arxiv.org/abs/0704.2928


 2. Nisse

- Title: Tropical geometry as limiting aspect of algebraic geometry.
- Abstract: The goal of this series of lectures is to provide a quick overview of tropical geometry  through some examples with the maximum of details.  After defining a  basic objects in tropical geometry, we explain their relations to the classical algebraic  geometry via the notion of  amoebas and coamoebas of algebraic varieties and their degenerations. We will give some applications of tropical geometry to real and complex enumerative geometry. 

- References:
(1) I. Itenberg, G Mikhalkin, and E. Shustin,  Tropical Algebraic Geometry, volume 35 of Oberwolfach Seminars Series. Birkh?user, 2007.
(2) D. Maclagan and B. Sturmfels,   Introduction to tropical geometry. Graduate Studies in Mathematics, Vol 161, American  Mathematical Society, Providence, RI, 2015. 
(3) G. Mikhalkin,   Enumerative tropical algebraic geometry in $mathbb{R}^2$. J. Amer. Math. Soc.,18, (2), 313?377, 2005.
(4) G. Mikhalkin,  Tropical geometry and its applications. In International Congress of Mathematicians. Vol. II, pages 827?852. Eur. Math. Soc., Zurich, 2006.
(5) M. Nisse  and F. Sottile,  The phase limit set of a variety,  Algebra & Number Theory, 7, (2013), 339--352.
(6) J. Richter-Gebert, B. Sturmfels, and T. Theobald,  First steps in tropical geometry. In Idempotent mathematics and mathematical physics, volume 377 of Contemp. Math., 289?317. Amer. Math. Soc., Providence, RI, 2005.