Workshop on

Liouville theory, Integrable system, and related topics

Title & Abstract

Home > Title & Abstract

Title & Abstract

- 3 hours Talk
Speaker: Marco Bertola (Concordia University)
Title: Tau functions for isomonodromic systems and general Riemann--Hilbert problems. Theory and applications.
Abstract: In this three hour course I will explain the main theory and properties of the above mentioned tau function. These special functions are in a certain sense a generalization of Riemann Theta functions; they can be also thought of as (regularized) determinants. In certain cases they are genuine (finite or infinite-dimensional) determinants. As such their vanishing determines the obstruction to the solvability of a certain linear problem.

The tau function was originally defined for isomonodromic systems (e.g. the associated linear systems for the Painlev'e equations) but then the definition can be extended to more general Riemann--Hilbert problems; this extension does not require a linear ODE. Even in the case of the ``isomonodromic'' tau function, this extension allows to determine consistently the dependence on the (generalized) monodromy data.

I will explain various applications to

1) integrable systems (KdV, Toda, KP)

2) Witten-Kontsevich special tau function and intersection numbers; generating functions.

3) Gap probabilities (Tracy-Widom and generalizations thereof); Fredholm determinants.

4) (Multi)-Orthogonal polynomials and Hankel/Toeplitz determinants. Random Matrices.

Speaker:Katsunori Iwasaki (Hokkaido University)
Title: Geometry and dynamics of Painlev'e equations
Abstract: After a general introduction to Painlev'e equations we focus our attention on the geometry and dynamics of the sixth
Painlev'e equation. My talk is divided into three parts. Part I concerns algebraic geometry of phase spaces of Painlev'e dynamics;
moduli space of parabolic connections, Riemann-Hilbert correspondence, etc. Part II is about dynamics of nonlinear monodromy maps of Painlev'e equation; ergodic theory, entropy, invariant curves, exponential growth of the number of periodic solutions, etc. where our emphasis is on chaotic aspects rather than integrable aspects. Part III develops a general theory of periodic points for area-preserving birational maps of surfaces, which is used in Part II. This talk is based on my joint works with M. Inaba and M.-H. Saito in algebraic geometry part and with T. Uehara in dynamical system part.

Speaker:Joerg Teschner (University of Hamburg)
Title: Liouville theory, classical and quantum isomonodromic deformations
The goal of my lectures will be to explain the known relations between Liouville theory and the theory of isomonodromic deformations.
Part 1 will introduce the building blocks for correlation functions in Liouville conformal field theory called conformal blocks, which can be defined using the representations theory of the Virasoro algebra.
The second part will describe relations to the theory of isomonodromic deformations arising in the classical limit, equivalent to the limit of infinite central charge. These relations allow us to regard Liouville theory as a quantisation of the theory of isomonodromic deformations.
Part 3 will be devoted to a recent result (joint work with N. Iorgov and O. Lisovyy) relating linear combinations of Liouville conformal blocks at unit central charge to tau-functions of the isomondromic deformation equations. If time permits we will discuss the deep relation of this result to (quantum) Teichmueller thery.

Speaker:Richard Wentworth (University of Maryland)
Title: Geometric and analytic aspects of moduli spaces of Higgs bundles
Abstract: The first part of this series of talks will be a quick introduction to the foundations of the theory of Higgs bundles on Riemann surfaces. Important among these are the hyperkaehler structure, spectral curve construction, and the relationship with differential equations and Teichmueller theory. In the second lecture I will present recent work with Francois Labourie on variational properties of dynamical quantities associated to Hitchin and oper representations, and to the symplectic structure along the Fuchsian locus. This work generalizes familiar results from classical Teichmueller theory. Time permitting, the goal of the last lecture will be to suggest an approach to the construction of a hyperholomorphic line bundle on the moduli space of Higgs bundles that is based on the Deligne isomorphism and holomorphic extension of analytic torsion. This latter part is joint work with Gerard Freixas i Montplet.

- 1 hour Talk
Speaker: Hyun Kyu Kim (KIAS)
Title: Quantum Teichmuller theory from representation theory of quantum groups
Abstract: The main result of quantum Teichmuller theory is an assignment of a unitary operator to every change of ideal triangulations on a punctured Riemann surface, so that the composition is preserved up to multiplicative constants. We notice that triangulations of a polygon correspond to choices of arrangement of parentheses of tensor products of finitely many objects. We show that the associativity morphisms of the tensor category of certain representations of a rather basic quantum group almost coincide with the operators of quantum Teichmuller theory. This is a joint work with Igor Frenkel.

Speaker:Alexey Kokotov (Concordia University)
Title: Moduli spaces of meromorphic functions and determinant of Laplacian.

Speaker:Dmitry Korotkin (Concordia University)
Title: Symplectic structure on periods of quadratic differentials on Riemann surfaces
Abstract: We introduce a natural symplectic structure in terms of homological coordinates on moduli spaces of quadratic differentials with simple zeros. This structure turns out to coincide with the canonical Poisson structure on the cotangent bundle over the moduli space of Riemann surfaces; therefore, homological coordinates give a new system of Darboux coordinates on the cotangent bundle. We consider various ways of inducing the canonical Poisson structure from the cotangent bundle to the space of projective structures over Riemann surfaces; this requires the use of a base projective connection which holomorphically depends on moduli. Chosing various projective connections of this kind (Bergman, Schottky, Wirtinger, Bers) we show that the corresponding symplectic structures on the space of projective connections are equivalent, and find the corresponding generating functions. We also study the monodromy map from the space of projective connections to monodromy representation of the Schwarzian equation, and show that the natural symplectic structure on the the space of projective connections induces Goldman Poisson structure on monodromies (this fact, being combined with results by S.Kawai,
show equivalence between Bers and Bergman projective connections).

Speaker:Andrew McIntyre (Bennington College)
Title: TBA

Speaker: Uhi Rinn Suh (Seoul National University)
Title: Equivalent definitions of affine classical W-algebras (and fractional W-algebras)
Abstract: There are 4 types of W-algebras, say affine classical, finite classical, affine quantum and finite quantum W-algebras. They have Poisson vertex algebra, Poisson algebra, vertex algebra and associative algebra structures, respectively. Hence natural constructions of affine classical W-algebras can be obtained via quasi-classical limits of affine quantum W-algebras and via chiralizations of finite classical W-algebas. Also, in the theory of Hamiltonian integrable systems, a W-algebra appears as an underlying algebraic structure of an integrable system. In this talk, I will show three of these constructions can be understood as equivalent definitions of affine classical W-aglebras.
Analogously, (If time allows) I will introduce fractional W-algebras which are generalizations of affine classical W-algebras and explain algebraic structures on them.

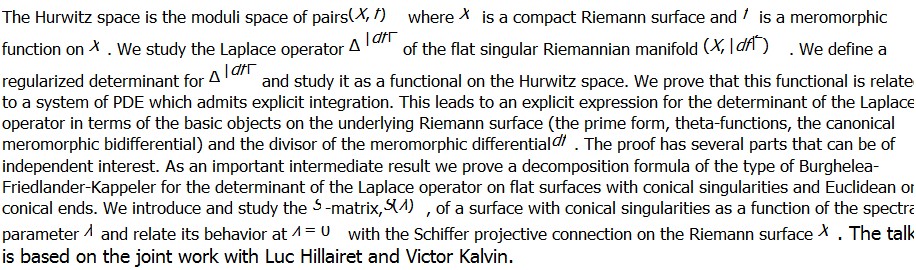
Speaker: Lee Peng Teo ( University of Nottingham Malaysia Campus)
Title: Liouville Theory and Index Theorem on Universal Teichmuller Space
Abstract: