Conference On Moduli and Birational geometry (COMB) V Period: Dec. 12~16, 2016 Venue: Haevichi Resort (Jeju, Korea) |
| Title & Abstract | Home > Title & Abstract |
Sebastian Casalaina-Martin (Colorado)
Title: The moduli space of cubic threefolds via intermediate Jacobians
Abstract: Associating to a smooth cubic threefold its principally polarized intermediate Jacobian induces a rational period map from the GIT moduli space of cubic threefolds to the second Voronoi compactification of the moduli space of five dimensional principally polarized abelian varieties. In this talk I will describe a resolution of the period map, which allows for a geometric description of the boundary of the moduli space of intermediate Jacobians. This is joint work with Samuel Grushevsky, Klaus Hulek, and Radu Laza.
Dawei Chen (Boston)
Title: Principal boundary of strata of abelian differentials
Abstract: Eskin-Masur-Zorich described the principal boundary of strata of abelian differentials that parameterizes flat surfaces with a prescribed generic configuration of short parallel saddle connections. As an application, they gave a recursive algorithm for calculating the associated Siegel-Veech constants. In this talk we describe the principal boundary algebraically over the Deligne-Mumford boundary of stable pointed curves. Along the way we deduce some interesting properties about meromorphic differentials on the Riemann sphere. This is joint work with Qile Chen based on arXiv:1611.01591.
Meng Chen (Fudan)
Title: On minimal 3-folds of general type with maximal pluricanonical section index
Abstract: Let $X$ be a minimal 3-fold of general type. The pluricanonical section index $delta(X)$ is defined to be the minimal integer $m$ so that $P_{m}(X)geq 2$. According to Chen-Chen, either $1leq delta(X)leq 15$ or $delta(X)=18$. This note aims to intensively study those with maximal such index. A direct corollary is that the 57th canonical map of every minimal 3-fold of general type is stably birational.
Anand Deopurkar (Georgia)
Title: Vector bundles and finite covers
Abstract: Let Y be a variety. A finite cover X -> Y of Y gives a natural vector bundle on Y, namely the direct image of the structure sheaf of X. Which vector bundles on Y arise in this way? I will present an answer to an asymptotic version of this question when Y is a curve, generalizing previous results of Ballico and Kanev, and answering a question of Lazarsfeld. This is joint work with Anand Patel.
Maksym Fedorchuk (Boston)
Title: Invariant theory of Milnor algebras
Abstract: I will discuss the interplay between hypersurface singularities, their Milnor algebras, and classical invariant theory of homogeneous forms. In particular, I will prove that a contravariant that associates to a smooth homogeneous form the Macaulay inverse system of its Milnor algebra preserves GIT stability. I will discuss some applications of this result, for example to the direct sum decomposability of polynomials, and many related open problems.
Victoria Hoskins (Berlin)
Title: Group actions on quiver moduli spaces
Abstract: We consider two types of actions on moduli spaces of quiver representations over a field k and we decompose their fixed loci using group cohomology. First, for a perfect field k, we study the action of the absolute Galois group of k on the points of this quiver moduli space valued in an algebraic closure of k; the fixed locus is the set of k-rational points and we obtain a decomposition of this fixed locus indexed by the Brauer group of k. Second, we study algebraic actions of finite groups of quiver automorphisms on these moduli spaces; the fixed locus is decomposed using group cohomology and each component has a modular interpretation. If time permits, we will describe some examples, such as the Hilbert scheme of point in the plane and polygon spaces, and give some applications to the construction of branes in hyperkaehler quiver varieties. This is all joint work with Florent Schaffhauser.
Jack Huizenga (Penn. State)
Title: Weak Brill-Noether for rational surfaces
Abstract: A moduli space of sheaves satisfies weak Brill-Noether if the general sheaf in the moduli space has no cohomology. Goettsche and Hirschowitz prove that on the projective plane every moduli space of Gieseker semistable sheaves of rank at least two and Euler characteristic zero satisfies weak Brill-Noether. We completely characterize Chern characters on Hirzebruch surfaces for which weak Brill-Noether holds. We also use combinatorial methods to prove that on a del Pezzo surface of degree at least 4 weak Brill-Noether holds if the first Chern class is nef. This is joint work with Izzet Coskun.
DongSeon Hwang (Ajou)
Title: Birational construction of log del Pezzo surfaces of Picard number one
Abstract: Every nonsingular del Pezzo surface is either the projective plane, the quadric surface, or the blow-up of the projective plane in at most $8$ general points. In particular, every nonsingular del Pezzo surface of Picard number at least three admits a surjective birational morphism to the projective plane obtained by composing the given blow-down morphisms.
In this talk, I would like to propose a new notion, called a cascade, which generalize the above birational construction mechanism for nonsingular del Pezzo surfaces to that for log del Pezzo surfaces of Picard number one. Secondly, I will show that every log del Pezzo surface of Picard number one admits a cascade and explain how it can be used to enumerate all log del Pezzo surfaces of Picard number one. The proof is done by analyzing the behavior of minimal curves on the minimal resolution of a log del Pezzo surface of Picard number one based on Miyanishi-Zhang's theory. If time permits, I will also remark on its relation with a conjecture called the algebraic Montgomery-Yang problem.
Masayuki Kawakita (RIMS)
Title: Divisors computing the minimal log discrepancy
Abstract: The minimal log discrepancy is an important invariant of singularities in the minimal model program but we do not have a good understanding of it. We will discuss the question which divisor computes the minimal log discrepancy. In particular, we will study the case in which such a divisor is extracted by a weighted blow-up.
Michael Kemeny (Stanford)
Title: Effective bounds for Green-Lazarsfeld's Gonality Conjecture
Abstract: In 1986, Green and Lazarsfeld conjectured that one can read off the gonality of a curve from the length of the linear part of the resolution of any embedding of the curve of sufficiently high degree. This was proven two years ago by Ein and Lazarsfeld, but the question of determining how large the degree of the embedding must be has remained open. We show that the solution to this problem is d >= 2g-1+k, under the assumption that the curve is general of prescribed gonality k. This result is optimal (both in the bound and in the sense that it fails for certain special curves).
Young-Hoon Kiem (SNU)
Title: Stability conditions on linear systems with p-fields
Abstract: A curve C in a projective variety X is given by a linear system on C, which consists of a line bundle L together with a sequence of sections satisfying the equations of X. When X is a quintic Calabi-Yau 3-fold, it is more useful to add a homomorphism, called a p-field, from the fifth power of L to the dualizing sheaf of C. Based on Le Potier's stability conditions, I will introduce two sequences of stability conditions which give us the Gromov-Witten invariant and the Fan-Jarvis-Ruan-Witten invariant as well as sequences of interpolating invariants. I will also discuss wall crossing and applications. This is based on a joint work with Jinwon Choi.
Zhiyuan Li (SCMS, Fudan)
Title: Tautological rings on moduli space of irreducible holomorphic symplectic varieties
Abstract: The tautological ring on moduli of K3 surfaces are recently introduced by Marian, Oprea and Pandharipande. There is a tautological conjecture which says that this ring is generated by Neother-Lefschetz cycles, In this talk, we extend this definition to moduli space of projective IHS varieties and prove the generalized tautological conjecture in cohomology. This is a joint work with N.Bergeron.
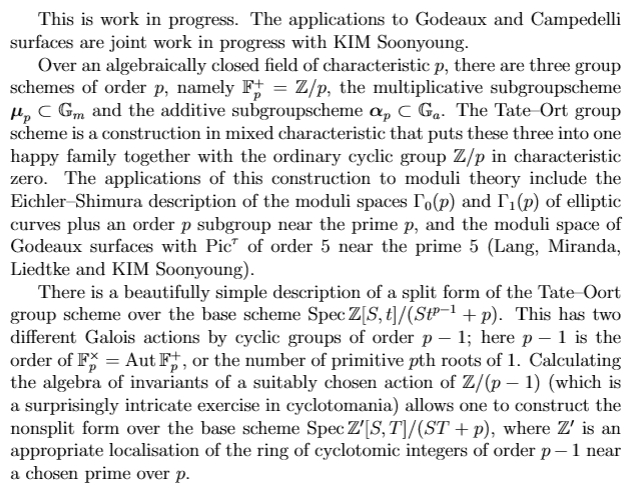
Miles Reid (Warwick / KIAS)
Title: The Tate–Oort group scheme of order p
Abstract:
David Swinarski (Fordham)
Title: Vector partition functions for conformal blocks
Abstract: A vector partition function is a function that counts the number of lattice points in a polytope defined by the function's arguments. It is conjectured that the ranks of vector bundles of conformal blocks on the moduli space of curves and the intersection numbers of their first Chern classes with F-curves are given by vector partition functions. I will discuss consequences of these conjectures and progress toward proving them.
Nicola Tarasca (Fields Institute, Toronto)
Title: Hyperelliptic loci in moduli spaces of curves
Abstract: In this talk, I will discuss some recent results in the enumerative geometry of subvarieties of moduli spaces of curves. In joint work with Dawei Chen, we study the extremality of loci of hyperelliptic curves with marked Weierstrass points inside cones of effective classes of high codimension. I will present a close formula for classes of loci of genus-two curves with marked Weierstrass points as result of an ongoing work with Renzo Cavalieri. These are among the first results toward the study of cones of higher codimensional effective classes.
Jenia Tevelev (Massachusetts Amherst)
Title: Derived category of the moduli space of stable rational curves
Abstract: I will discuss work in progress with Ana-Maria Castravet verifying a surprising conjecture of Orlov and Kuznetsov on the equivariant structure of the derived category (and even K-theory) of the moduli space of stable rational curves.
Filippo Viviani (Rome Tre)
Title: The cohomology of the Hilbert scheme and of the compactified Jacobians of a singular curve
Abstract: We generalize the classical MacDonald formula for smooth curves to reduced curves with planar singularities. More precisely, we show that the cohomologies of the Hilbert schemes of points on a such a curve are encoded in the cohomologies of the fine compactified Jacobians of its connected subcurves, via the perverse Leray filtration. The proof uses the equigeneric stratification of the semiuniversal deformation space of the curve to reduce to the case of nodal curves, where everything can be computed explicitly.
This is a joint work with Luca Migliorini and Vivek Schende.
